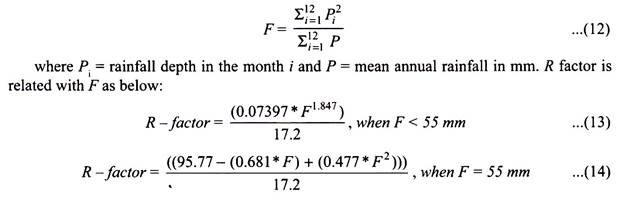ADVERTISEMENTS:
Presently, there are several tasks in hydrology, which need application of suitable models for their accomplishment. To decide suitability of a particular model, first the user should look at the availability of the data and model requirements, and then, a suitable model should be chosen for performing the task.
Few of the hydrological tasks are listed below where modeling has been successfully applied for determining some hydrologic parameter/variable for subsequently used for soil and water resources management:
1. Peak rate of runoff for designing water resource management structures,
ADVERTISEMENTS:
2. Total annual water yield from a catchment
3. Probable peak flood and time of flood after certain storm
4. Annual sediment yield from a defined catchment
5. Estimating storage capacity of a water body to be created
ADVERTISEMENTS:
6. Estimating groundwater potential in the given area
7. Groundwater recharge in terms of both volume and depth
8. Crop water requirements
9. Water balancing
ADVERTISEMENTS:
10. Advances of floods
11. Channel flow and hydro-dynamic modeling
Of the several hydrological tasks, hydrological modeling tools for computing runoff from a catchment and for estimating sediment yield are discussed and demonstrated by applying the procedures to case studies of the drylands.
1. Rainfall-Runoff Modeling:
Runoff from a watershed/catchment system is usually assessed by analyzing the data of gauging stations installed at the catchment outlet. However, due to high costs and manpower involved in continuous data recording, it is not always feasible to have a long-term runoff database.
ADVERTISEMENTS:
Alternatively, one uses a prediction model for gauged catchment or apply a suitable runoff estimation procedure for ungauged catchments. In India, majority of the agricultural and forest catchments especially in dry areas are ungauged, having no past record whatsoever of rainfall-runoff processes.
The initial step for developing a model is the conceptualization. The conceptualization of a typical water balance model is presented. Out of several methods for runoff estimation from ungauged catchment, the Natural Resources Conservation Services Curve Number (NRCS-CN) method along with its derivatives have been widely applied to ungauged catchment systems and proved to be a quicker and accurate estimator of surface runoff. This method is widely used in applied hydrology. Although, this method was originally developed for its use on small agricultural watershed, but has since been extended and applied to rural, forest and urban catchment systems in many parts of the world including India.
The watershed hydrologic responses leading to generation of surface runoff are governed by the interaction of precipitation with the topological, land use and soil physical properties of the land surface. Therefore, use of Geographic Information System (GIS) is preferred over the traditional techniques for proper quantification of surface runoff by storing and analyzing the causative factors responsible for runoff generation.
Procedures for estimating peak runoff rate and total runoff yield from a catchment are explained here:
ADVERTISEMENTS:
a. Peak Rate of Runoff by Rational Model:
An important method for determining the peak runoff rate is the rational formula.
It is an empirically developed model, characterized by consideration of the entire drainage area as a single unit and estimation of flow at the most downstream point with the following assumptions-
(i) Rainfall is uniformly distributed over the drainage area for the duration equal to time of concentration,
(ii) The predicted peak discharge has the same probability of occurrence (return period) as the used rainfall intensity (I),
(iii) The runoff coefficient (C) is constant during the rain storm, and
(iv) The recession time is equal to the time of rise or time of concentration.
The expression for the rational formula is given as follows:
Qp= 0.28 × C× I × A … (1)
Where, Qp= peak runoff rate (m3 s-1), C = runoff coefficient, I = rainfall intensity (mm hr -1), and A = drainage area (km2).
I. Methodology:
(i) The rational formula uses the runoff coefficient (C), which is related to the different land use/land covers and hydrologic soil groups. In general, a catchment has more than one land use/land cover types with presence of more than one soil group. Therefore, weighted value of the representative/overall runoff coefficient is required to be determined using the areas of the different land use/land cover and hydrologic soil group complexes as weighting factor.
The weighted CN is computed from the following equation:
Where, CN weighted = weighted curve number for the entire catchment under AMC II conditions; CNi = curve number for ith combination/polygon of land use/land cover and HSG; Ai = area of ith polygon; and n = total combinations of the land use/land cover and HSG.
(ii) Isopluvial maps of different return periods and duration of storms can be used to get information of rainfall intensity for the desired return period. Thus, it requires the inputs of return period and outlet point for computing the peak rate of runoff of a storm. Then, peak rate of runoff can be determined for the return period defined by user.
II. Thematic Layers Needed for GIS Modeling:
(i) Digital Elevation Model (DEM)
(ii) Isopluvial maps of different return periods and durations for computing desired rainfall intensity
(iii) Land use/land cover layer
(iv) Hydrologic soil group layer
b. Total Runoff Yield by SCS-CN Model:
The SCS-CN model is based on the water balance equation and two fundamental hypotheses. The first hypothesis equates the ratio of the amount of direct surface runoff (Q) to the total rainfall P (or maximum potential surface to the runoff) with the ratio of the amount of infiltration (Fc) to the amount of the potential maximum retention (S). The second potential equality hypothesis relates the initial abstraction (Ia) to maximum retention (S).
Thus, the SCS-CN method consists of the following equations:
i. Methodology:
A flowchart illustrates step-by-step methodology for computing annual surface runoff by using remote sensing and GIS techniques are shown in Fig. 4.3.
ii. GIS-Based Thematic Layers Needed for Modeling:
(i) Land use/land cover layer
(ii) Hydrologic soil group layer
(iii) Hydrological soil group classification table
(iv) Curve number classification table for Indian conditions
2. Soil Erosion Modeling:
For most of the world’s regions accurate estimates of soil erosion are not available. Hence, development and application of a GIS-based soil erosion prediction methodology is of utmost importance. The Universal Soil Loss Equation (USLE) and GIS interface provides an effective tool for modeling soil erosion on regional basis.
The soil erosion prediction models other than USLE ranging from simple to complex are also available, which can provide more accurate estimates of soil erosion for specific sites. The choice of a suitable method depends upon the user’s wish depending upon the specific data requirements of those models and their applicability to use on a regional scale. By comparison, data for utilization of USLE can be relatively easy to obtain.
The USLE model was developed based on 40 years experimental field observations of Agricultural Research Service of the United States Department of Agriculture, and presently, it is the most-widely used soil erosion assessment model. The USLE model is considered as an index method associating factors that represent how climate, soil, topography, and land use affect rill and inter-rill soil erosion caused by rain drop impact and surface runoff. The USLE model determines the soil loss for a given area as a product of six key factors whose values at a particular location can be expressed numerically.
The soil loss can be computed by using the following USLE model expressed as:
A = R × K × LS ×C × P … (11)
Where, A = average annual soil loss (tonnes ha -1 year -1); R = rainfall erosivity factor (MJ mm ha-1h -1 year-1); K = soil erodibility factor (tonnes ha h ha-1MJ-1 mm-1); L – slope length (dimensionless); S = steepness factor (dimensionless); C = cover and management factor (dimensionless); and P = support practice factor (dimensionless).
In the modeling procedure, the values for the different USLE factors are assigned to their respective mapping units, i.e. a soil unit map, a vegetation class map, and a digital elevation model. Values for the factors can be obtained from reference sources or calculated from mapped or field collected data about the project location.
The rainfall erosivity factor (R factor) is defined as the product of total storm energy and maximum 30-min intensity divided by 100 for numerical convenience, known as the EI30 index. The EI30 index method is developed by evaluating correlations between soil erosion and a number of rainfall parameters. The annual R factor is computed as sum of EI30 values for individual storms during a year.
If rainfall intensity data are not available, Fournier Index and R factor can be calculated for desired catchment:
R factor map layer can be prepared in GIS after getting the values of different stations.
The K factor, termed as ‘soil erodibility factor’, is the integrated effect of processes that regulate rainfall acceptance and resistance of soil to particle detachment and transportation. The K factor is an empirical measure of soil erodibility and is a function of soil intrinsic properties. The main soil properties affecting the K factor are soil texture, amount of fine sand in addition to the usual sand, silt and clay percentages used to describe soil texture, organic matter, soil structure, and permeability of soil profile.
The K factor can be computed by empirical method, nomograph or K value triangle based on soil texture. The K factor values are obtained from soil surveys, or calculated from field information.
The preferred method, according to Goldman et al. (1986), for determining K factor is the nomograph method based on the work by Wischmeier et al. (1971), and is mathematically represented as follows:
Where,
M= particle size diameter,
a = organic matter percentage,
b = soil structure code, and
c = profile permeability class.
The slope length and steepness factor or LS factor is a product of two separate factors- slope length (L) and steepness (S). The slope length is defined as the horizontal distance from the point of origin of overland flow to either the point where the slope decreases sufficiently for deposition to begin or the point where runoff water enters a well-defined channel.
The LS factor is the most difficult parameter of USLE model to compute, and several methods have been devised to compute the LS from complex topographic terrain. The slope gradient S and slope length L factors are calculated from digital elevation data using GIS hydrologic terrain analysis capabilities and map algebra.
The L-S factor in USLE reflects the effect of topography on erosion and can be calculated as follows:
Where, As = specific watershed area/unit contributing area (m2/m), β = slope angle in radians and m (0.4-0.56) and n (1.2 – 1.3) are exponents. The raster calculator tool can be used for calculating L-S factor in GIS.
The crop and management factor (C factor) has marked effect on soil erosion and runoff by different type of vegetation and cropping systems. The C factor is described as the ratio of soil loss from land cropped under specified conditions to the corresponding loss from clean-tilled, continuous fallow land.
The effectiveness order of different vegetation and cropping systems is undisturbed forests > dense grass > forage crops (legumes and grasses) > small grains (wheat, oats, etc.) > row crops (corn, soybean, potatoes). The C factor can be related to normalized difference vegetation index (NDVI) with the following expression-
The P factor reflects the impact of support practices dealing with the average annual erosion rate. The support practice factor (P factor) is defined as the ratio of soil loss with a specific support practice to the corresponding soil loss with up and down cultivation.
The lower the P value, the more effective the conservation practice is deemed to be at reducing soil erosion. In absence of any support practices, the P factor is 1.0 (highest). The erosion control P factor values are obtained from some standard literature based on characteristics of particular area.
After the factor values are assigned or calculated for each of the mapping units, the factor maps are overlaid to produce a visualization of soil erosion potential.
b. Thematic Layers Needed for GIS-Based Soil Erosion Modeling:
(i) Monthly and mean annual rainfall
(ii) Information about soil properties, i.e. texture, organic matter and permeability, etc.
(iii) Digital elevation model
(iv) Vegetation in the form of Normalized Difference Vegetation Index (NDVI)
(v) Land use/land covers information with the available management practices