ADVERTISEMENTS:
After reading this article you will learn about the estimation of soil loss caused by water erosion.
While direct measurement of soil loss from an agricultural watershed is desirable, in many instances for example in determining adequacy of conservation measures in farm planning, the prediction of soil loss for a given set of conditions may be sufficient. However, the method used for predicting soil loss should consider each of the factors involved and be easily applied to field conditions.
Ellison (1945) showed the effect of rainfall energy in sheet erosion by the equation:
ADVERTISEMENTS:
E = Kv4.33d1.07i065 …(2.7)
where:
E = grams of soil intercepted in splash sampler during a 30 min period
v = drop velocity, ft./sec
ADVERTISEMENTS:
d = diameter of the drops, mm
i = intensity of rainfall, inches/hour
K = constant
Ellison’s model was later modified by Musgrave (1947) who incorporated land characteristics in the equation as:
ADVERTISEMENTS:
E = IK Rc S1.35 L0.35 P1.7530 …(2.8)
where, E = soil loss, acre inches
IK = inherent erodibility of soil, inches
Rc – cover factor
ADVERTISEMENTS:
S = degree of slope, %
L = length of slope, ft.
P30, = 2 years, 30 min rainfall amount, inches
The Musgrave equation has been widely used for estimating gross erosion from large heterogeneous watersheds. Its highly generalized factor values are more easily assigned to broad areas than for factors based on more specific descriptions of the erosion influencing parameters.
ADVERTISEMENTS:
The most accurate soil loss equation that is now field operational is the universal soil loss equation developed by Smith and Wischmeier (1957, 1962). The equation is most comprehensive and includes six variables important in soil erosion by water.
Xa = RKLSCP …(2.9)
where:
Xa = average annual soil loss t/acre
R = erosive potential rainfall factor of the average annual rainfall as given by the ‘Erosion Index’
K = the soil erodibility factor
L = the slope length factor
5 = the slope steepness factor
C = the cropping-management factor
P = the conservation practice factor
Some description of the terms in Eq. 2.9 along with factors associated with them has been detailed below:
Rainfall Factor (R):
From the assembled plot data, it was observed that when factors other than rainfall are constant, storm soil losses from a cultivated field are directly proportional to an interaction term viewed as rainfall factor. The rainfall factor, R, is the product of kinetic energy E, of the storm and its maximum 30 minute intensity and is called the rainfall Erosion Index (EI).
The term rainfall erosion index implies to a numerical evaluation of a rainstorm or of a rainfall pattern which describes its capacity to erode soil from an unprotected field. Differences in rainfall erosion potential are not necessarily associated with comparable differences in rainfall amount.
The various intensities involved in a specific rain, antecedent climatic and surface conditions, interaction effects and extraneous variables all influence the erosion potential of a storm.
The rainfall factor, R, is expressed as:
R = Total rainfall energy (E) x I30/100 …(2.10)
where, I30 = 30 min. maximum rainfall intensity for each storm, and
E = 916 + 331 log i, for / > 0 …(2.11)
where, E = kinetic energy in ft. – t/acre-inch of rain
i = rainfall intensity, inches/hour
The computation of storm rainfall energy as one component of EI value requires exact definition of an ‘individual storm’. The optimum minimum time to define as a break between storm is a function of the change in infiltration rate after cessation of a rain and, therefore, varies with soil type.
In general, the best correlations of soil loss amounts and EI values have been obtained when the rain separated by less than 6 hr are treated as a single storm.
The relation of soil loss to EI is linear, therefore, individual storm values of EI can be summed to obtain seasonal or annual values of the parameter. The energy of a rainstorm is a function of all its component intensities and rainfall amounts and can be computed from recording-rain gauge data.
The storm is divided into successive increments of essentially uniform intensity and the energy of each increment is computed using Eq. 2.11 or the rainfall energy-intensity table derived from Eq. 2.11 as given below (Table 2.3). Annual total of storm EI value is referred as the rainfall erosion index. For a particular location, annual values of erosion index follow a log normal distribution.
Recent studies have shown that mean drop size does not continue to increase when intensities exceed about 7.5 cm/hr. Therefore, the energy given in Table 2.3 for a 7.5 cm/hr intensity may be used for all higher intensities as well.
For computation of average annual EI values, continuous records from 20-22 years are desirable in order to avoid bias by cyclical variation in rainfall patterns. The computed annual EI values are also found to correlate reasonably well with 2 years, 6 hour rainfall probabilities.
The relationship has been expressed as:
EI = 27.38 P2.17 …(2.12)
where, P = 2 year, 6 hr rainfall
Estimates have shown that there are at-least two conditions for which the computed EI must be modified to evaluate the factor R. One, where snow melt runoff on moderate to steep slopes is significant, the EI value must be adjusted upward to add the erosive effects of this runoff to the R value. Two, the value of factor R for the coastal plains has been less than the EI values computed by the standard procedure.
This discrepancy may be due to the combination of hurricane-associated storms and flat slopes. The hurricane storms compute very high EI values, but the gentle slopes are soon largely covered by very slowly moving runoff that shields the soil surface from the raindrop impact.
Soil Erodibility Factor K:
The soil erodibility factor K refers to the soils inherent susceptibility to erosion by rainfall and runoff. This is a function of complex interactions of soil physical and chemical properties affecting detachability, transportability and infiltration capacity. It is expressed as average loss in t/acre/unit of erosion index for a particular soil of a unit plot.
The unit plot is defined as cultivated-continuous-fallow plot with an arbitrarily selected slope length L of 22 m and slope steepness S of 9%. Continuous fallow is defined as land which has been tilled, kept clear of vegetation for a period greater than three years.
Since erodibility represents the vulnerability or susceptibility of the soil to erosion, this is the most important fundamental property, dependent upon soil type. Observations indicate that erodibility decreases with increase in grade and size of clods, coarseness of texture, amount and size of coarse fragments, organic matter content and water intake capacity of the soil. K is the only factor in Eq. 2.9 which has dimensions.
Since detachability, transportability and infiltration capacity combine to make up the erodibility of a soil, each of these three factors have to be studied. Detachability may be determined in the field using splash boards or in the laboratory by artificial rain on soil samples in cylinders. In both the cases, amount of soil splashed from a given area per unit time is measured.
Transportability is estimated by determination of the proportion of soil particles of clay or silt size that can be suspended in water by gentle shaking. The opposite of this, the percentage of water-stable aggregates, can also be determined for this purpose.
The infiltration capacity can be measured directly on natural watersheds with the help of in-filtrometers. Erodibility is also affected by the slope. The relative erodibility of two soils may be reversed by changing the slope of the land.
Since very little data are available from plots of 22 m long on 9% slope and under continuous fallow for estimation of K, following expression has been suggested (Olson 1961) to determine K based on available data from runoff plots of different sizes.
K – X’a/Total EI …(2.13)
where,
Xa = Total expected soil loss from a fallow plot 22 m in length and 9% slope;
X’a can be calculated from:
X’a = X0/LSCP …(2.14)
where X0 = observed soil loss from the plot.
Using Eqs. 2.13 and 2.14 and data of bare runoff plots on 8% slope, the value of K has been found to vary from 0.28 to 0.36 tonnes/ha/EI at Dehradun. The use of an average value of 0.30 is recommended for Dhulkot silty loam at Dehradun.
In lateritic soils of Ootacamund the value of K has been found to be 0.04 tonnes/ha/EI from the data of runoff plots on 25% slope and 11 m length. Based on the particle size distribution, organic matter content, soil structure and permeability the following equation for predicting the inherent soil erodibility has been suggested.
K – (2.1 x 10-6) (12-Om) M1.14 + 0.0325 (S – 2) + 0.025 (P – 3) …(2.15)
where, Om = percent organic matter
M = particle size parameter [% silt (100% clay)]. Here the very fine sand is included in the silt fraction
S = structure index
P = permeability class, a profile parameter.
Topographic Factor LS:
The term LS are collectively known as topographic factor and given to adjust the soil loss from the standard length of 22 m and 9% slope to actual field.
These factors can be calculated from the relations:
L = (l/73)0.5 …(2.16)
and
S = 0.043 s2 + 0.30 s + 0.43/6.613 …(2.17)
where, l = slope length, ft.
s = field slope, per cent
The product LS can also be read directly from Fig. 2.13. Soil loss per unit area increases as slopes become longer or steeper. Both the terms are dimensionless and expressed relative to the “unit plot” dimensions defined for K factor.
Slope length is the distance from the point of origin of overland flow to the point where either the slope decreases enough that deposition begins, or the runoff water enters a well-defined channel. The effect of slope length is primarily due to greater accumulation and more channelization of runoff on the longer slopes.
This increases the capability of the runoff to detach and transport the soil material. For slopes steeper than 4% the factor L is generally computed by the above formula (Eq. 2.16). The exponent is 0.3 for slopes of less than 3% and 0.4 for 4% slopes.
Slope length is the distance from the point of origin of overland flow to the point where either the slope decreases enough that deposition begins, or the runoff water enters a well-defined channel. The effect of slope length is primarily due to greater accumulation and more channelization of runoff on the longer slopes.
This increases the capability of the runoff to detach and transport the soil material. For slopes steeper than 4% the factor L is generally computed by the above formula (Eq. 2.16). The exponent is 0.3 for slopes of less than 3% and 0.4 for 4% slopes.
Slope steepness affects both runoff and soil loss. In the assembled plot data, runoff from small area tended to increase linearly with increases in the slope. Shape of the slope is also important. When the slope steepens or flattens significantly toward the lower end or is composed of series of convex and concave segments, its overall average gradient and length do not correctly indicate the topographic effect on soil loss.
An irregular slope can be viewed as a series of segments such that the gradient within each segment can, for practical purposes, be considered uniform. The segments cannot be evaluated as independent slopes when runoff flows from one segment to the other.
Cropping Management Factor C:
The cropping management factor C is the ratio of soil loss for given conditions to soil loss from cultivated continuous fallow on identical soil, and slope and under the same rainfall. The cropping management factor includes the effect of cover, crop sequence, productivity level, length of growing season, tillage practices, residue management, and the expected time distribution of erosive rainstorms.
If the actual soil loss equals the potential loss predicted by the product of factors R, K, L and S; factor C = 1. This would be clean-tilled continuous fallow or land where mechanical de-surfacing has removed all the surface vegetation and most of the root zone.
Where, there is any vegetative cover or where the upper layer of soil contains significant amounts of roots or plant residues, or where cultural practices increase infiltration and reduce runoff velocity, soil loss is less than the product RKLS. Factor C brings this reduction into the soil loss computation. On cropped land C ranges from 1.0 to less than 0.01 (Tables 2.4 and 2.5).
The soil loss from continuous row crop, such as corn has been given the value of 1.0. If soil loss from continuous row crop is 12 tonnes/acre and the loss from oats in rotation is 3 tonnes/acre, then the C factor for oats is 0.25.
Land treatments that increase infiltration and the capacity of the soil to store water will reduce small watershed flooding that results from short, intense rains during the growing season. However, when the soil becomes saturated to a considerable depth, as is often the case in major flood periods, cultural practices have much less effect on the runoff.
The effect of cover and management on soil detachment and transport by rainfall and runoff are numerous and varied. The ension equation’s empirically determined factor C combines all of these effects in one numerical evaluation.
Extension of this factor to untested situations is facilitated by separating its total influence into three distinct types of effects and evaluating each type as a sub-factor:
(a) Effects of canopy cover;
(b) Effects of mulch or close-growing vegetation in direct contact with the soil surface; and
(c) Tillage and residual effects of the land use.
(a) Canopy cover:
Leaves and branches that do not directly contact the soil are effective only as canopy cover. Canopies have little influence on the amount and velocity of runoff from prolonged rains, but they do intercept falling raindrops. Water drops falling from the canopy may regain appreciable velocity, but usually less than free falling raindrops.
Therefore, the canopy reduces rainfall erosivity by reducing its impact energy at the soil surface. The amount of reduction depends on the height and density of the canopy. This effect can be viewed as a reduction in the effective EI of rainstorms, and can be directly computed for specific situations.
As shown in Eq. 2.2 the kinetic energy of a given mass is directly proportional to the square of its velocity. For example, a 2.5 mm drop falling from a height of 2 m would have a velocity of 5.19 m/sec. and only half the kinetic energy of a free falling raindrop of equal size.
Since the interception by canopy does not appreciably affect the/component of the EI parameter, the reduction in effective EI is directly proportional to the reduction in impact energy.
For partial canopies, ‘percent cover’ is defined as the percentage of the total surface area that could not be hit by vertically falling raindrops because of the canopy. Here, the effective EI for a given area is linearly proportional to the percentage of the ground that is not covered by the canopy. Influence of vegetal canopy on effective EI (Fig. 2.14) shows that for a 60% canopy cover at a height of 1 m, the canopy factor is 0.58.
This means that the effective EI with the canopy is only 58% of the actual EI of the rainfall, and the expected erosion would also be only 58% of that predicted by EI obtained from the iso-erodent map. Figure 2.14 is based on a medium drop size of 2.5 mm for both the rain and droplets formed on the canopy.
Cultivated legumes, such as cowpea, mung, urd, groundnut, guar and soya bean provide better crop cover and hence better protection to cultivated land against erosion than clean cultivated crops.
(b) Mulch and Close-Growing Vegetation:
A mulch on the surface is much more effective than an equivalent percentage of canopy cover.
There are two reasons for this:
(i) Since the intercepted rain drops have no remaining fall height to the ground, their impact on the soil surface is essentially eliminated;
(ii) A mulch that makes a good contact with the ground also reduces the velocity of runoff and therefore, greatly reduces the runoff potential to detach and transport soil material. Khybri (1979) reported that a grass mulch @ 4 t/ha applied in inter-rows of maize crop grown on 8% slope in Dhulkot silty clay loam, reduced runoff by 31% and soil loss by 37 t/ha.
Based on the rainfall simulator data, relationships have been developed to show the effect of per cent of soil surface cover by mulch on C factor. If the cover includes both canopy and surface mulch, the canopy and mulch factors overlap and cannot be fully credited because the impact energy of a raindrop that strikes the mulch is dissipated at that point regardless of whether canopy interception has reduced its fall velocity.
Here, the mulch factor is taken at full value and the canopy factor is reduced to apply only to the percentage of the surface not covered by mulch. For example, in a 30% mulch cover combined with a 60% canopy at a height of 1 m the factor for mulch cover effect is 0.47 (Fig. 2.15).
Because of 30% mulch cover, the effective canopy cover is only 0.70 of the overall 60% cover or 42% canopy cover, we obtain a factor of 0.70 for canopy effect. The factor for the combination of canopy and mulch; over is the product of the two sub-factors 0.47 and 0.70 or 0.33.
(c) Residual Effects of Land Use:
This category includes residual effects of the land use on soil structure, organic matter content and soil density, effects of tillage or lack of tillage on surface roughness and porosity, roots and sub-surface systems, biological effects, etc. The residual effect of land use on C-factor is shown in Fig. 2.16.
Smith and Wischmeier (1962) reported the erosion control effectiveness of each crop on the basis of five crop stage periods and the amount of erosive rain expected during each period as a ratio of the soil loss from continuous fallow (Table 2.6).
The Conservation Practice Factor P:
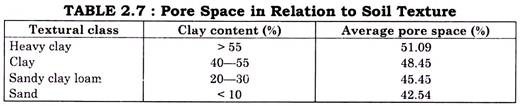
The conservation practice factor is the ratio of soil loss for a given practice to that for up and down the slope farming. The value of P for various practices such as contouring, strip cropping, and terracing arc given in Table 2.7. The factor for up and down slope is considered as 1.0. The reduction in soil loss at a given slope is about 50% of the next more intensive practice.
This factor is similar to C except that P accounts for additional effects of practices that are superimposed on the cultural practices, such as contouring, terracing, diversion and contour strip cropping, etc.