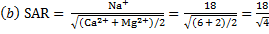Predicting the Annual Rate of Soil Loss: 11 Models | Soil Management

The following points highlight the eleven major models used for predicting the annual rate of soil loss. The models are: 1. Standford Sediment Model 2. Morgan, Morgan and Finney Model 3. F.A.O. Provisional Model 4. Stehlik Model 5. Slemsa Model 6. Erosion Deposition Process Model 7. Simplified Erosion Process Model 8. Water Erosion Prediction Project (WEPP) Model and Few Others.

1. Standford Sediment Model:

This model includes rainfall, overland flow and channel flow as input parameters, and the sediment removed from the hill slopes by overland flow and sediment derived from rill, gully and channel erosion are accounted as the output parameters. The operation of model is based on several functions, describing the process of soil erosion, expressed by several equations. The model is best illustrated by the flow chart, shown in Fig. 21.6.

The model for assessment of soil erosion, includes following three components:

1. Runoff component

2. Erosion component; and

3. Routing component.

The runoff component estimates the volume of overland flow. It is used as an input parameter to soil erosion component and the flow, contributing to the river/channel.

The erosion component computes the amount of sediment entering the river system from the hill slopes. This component also involves the erosion process such as detachment and transportation of sediments.

The routing component comprises the events of water movement and sediment flow through the river/channel system.

2. Morgan, Morgan and Finney Model:

Morgan et.al (1984) developed this model by compiling the results of various researches conducted by geomorphologists and agricultural engineers. This model can be used for predicting the annual soil loss from field-size areas on hill slopes.

The model includes total fifteen input parameters and six operating functions, given in Table 21.14 and 21.15, respectively. Model is divided into two phases for describing the soil erosion mechanism.

These phases are:

(a) Water phase and

(b) Sediment phases

(a) Water Phase:

It includes annual rainfall (R) as a basic input parameter. The rainfall energy (E) is computed by determining the energy of a typical intensity of rainfall, responsible for erosion. The equation given by Wischmeir and Smith (1958) can be used for calculating the E. The energy is multiplied by the annual rainfall amount, as shown in Table 21.15.

For calculation of E, the following values of rainfall intensity can be taken:

(i) For temperate climate: 11.0 mm/h.

(ii) For tropical climate: 25.0 mm/h.

(iii) For strongly seasonal climate: 30 mm/h.

(However, if the informations on typical intensities of erosive rainfall of the area is already available, then preference must be given to use that.)

In water phase the annual volume of overland flow is determined with the annual rainfall data, using the equation given by Carson and Kirkby (1972) shown in Table 21.15. The equation assumes that the runoff takes place, particularly when magnitude of total daily rainfall is greater than the critical value, which basically represents the moisture storage capacity (R_c) of the soil.

The value of R_c is obtained by using the equation described by Withers and Vipond (1974), which is based on the soil parameters such as soil moisture content (MS), bulk density (BD) and rooting depth (RD). The ‘R_c‘ obtained so, is adjusted for the effects of evapotranspiration following the methodology adopted by Kirkby (1976). The values of different soil properties needed to determine R_c are given in Table 21.16. Similarly, the values of E_t/E_o for different crops are also noted in Table 21.17.

(b) Sediment Phase:

It is simplified form of the model describing the process of soil erosion, given by Meyer and Wischmeir (1969). The flow chart of the model is shown in Fig. 21.7. This model assumes that the soil erosion is resulted from the impact of raindrop causing soil detachment and the detached particles are transported by the overland flow occurring during the rainfall, only.

The detachment as well as transportation of soil particles by runoff is not considered. The sediment phase comprises two predictive equations. One is for predicting the rate of soil detachment due to splashing, and second is for transport capacity of the overland flow. The input parameters for these equations are derived from the water phase.

The equation/model for soil detachment is the function of rainfall energy, incorporates the power relationship given by Meyer (1981 a) as shown in Table 21.15. In this equation the values of a & b are taken as 0.05 and 1.0, respectively.

The equation is modified to incorporate the effect of rainfall interception by the crops, by making assumption that the rainfall energy gets reduced exponentially with increase in interception. However, the typical values of interception of different crops for use in the model are also cited in Table 21.17.

The model for predicting the transport capacity of overland flow is formulated with the help of equation given by Kirkby (1976) and also by accounting the slope inclination and the effect of crop cover lying over the soil surface, as shown in Table 21.17. This equation is similar to the equation developed by Morgan (1990 a) given as –

For incorporating the effect of soil conservation practices on soil erosion, a separate phase can also be formed. For example, the effect of agronomical measures on erosion control can be introduced in terms of change in evapotranspiration, interception and crop management affecting the volume of overland flow/surface runoff, and thereby the soil erosion, too.

For determining the annual rate of soil loss, the model compares the predictions of splash detachment and transport capacity of the overland flow. The lower value of these two processes is counted as the annual rate of soil loss.

Limitations:

The main limitations of this prediction model are given as under:

1. This model is very sensitive to change in the annual rainfall and soil parameters, when erosion is transport-limited; and also sensitive to change in rainfall interception when erosion is detachment- limited.

2. It requires precise informations on rainfall and other associated parameters for accurate prediction.

3. This model cannot be used for calculating the sediment yield from drainage basin.

4. Like USLE, it is also not suitable for predicting the soil loss from an individual storm.

Soil Loss Prediction:

The procedure for predicting the soil loss, using Morgan et al (1984) model is little different than the Universal Soil Loss Equation. In this method, the rate of soil detachment by raindrop and transport by overland flow are computed; and lower value between these two is considered as the mean annual rate of soil loss.

3. F.A.O. Provisional Model:

The F.A.O. erosion prediction model is given below:

D = F (C.S.T.K) … (21.48)

Where,

D = soil degradation, i.e. soil erosion (t/ha/y)

C = climatic factor, i.e. rainfall in terms of yearly total

S = soil factor

T = topographic factor

K = constant, which represents the standard condition of natural vegetations, land use and management practices.

The soil loss is obtained by multiplying all the factors, associated to the equation.

The different factors are described as under:

i. Climatic Factor (C):

In equation 21.48, the climatic factor is related to the amount of annual rainfall received by the area. The values of ‘C’ for different ranges of rainfall are cited in Table 21.18.

ii. Soil Factor (S):

It refers to the soil erodibility factor, which is determined by taking into account the erodibility class and soil textural class, which are decided on the basis of soil mapping units, prepared at the scale 1:5 million FAO/UNESCO World Soil Map. The imputed values of ‘S’ for different soil erodibility classes and textural classes are given in Table 21.19 and 21.20, respectively. The derived values of soil erodibility factor (S) from both the tables, are multiplied to get composite value of soil erodibility.

iii. Topographic Factor (T):

The topographic factor is determined on the basis of dominant slopes with their categories. The imputed values are presented in Table 21.21.

The F.A.O. has classified the extent of soil erosion in different classes as per annual soil loss, given in Table 21.22.

(About F.A.O. erosion prediction equation a detail description has been given in the F.A.O. Bulletin, 1979; the readers can refer this for detail information.)

Limitations:

The important limitations of this equation, pointed by different researches are given as under:

1. The model is based on scientific principles and empirical data. The mathematical relationships used in this model are not exact representation of what is actually happening in the nature, but approximate effects of various environmental factors on soil erosion.

2. This model predicts an approximate value of soil degradation in terms of soil erosion.

3. The value of rainfall erosivity factor is based on the amount of annual precipitation without accounting their intensity.

4. The value of constant ‘K’ for natural vegetations, land use and management practices, requires a careful evaluation. Its application should be made according to the type of crops grown and natural vegetations.

4. Stehlik Model:

Stehlik (1975) devised this model for predicting the annual rate of soil loss in Czechoslovakia, which is given as under –

X = D.G.P.S.L.O. … (21.49)

Where,

X = mean annual soil loss (mm/year)

D = climatic factor (mm/year)

G = penological factor (dimensionless)

P = soil erodibility factor (dimensionless)

S = slope steepness factor (dimensionless)

L = slope length factor (dimensionless)

O = vegetation factor (dimensionless)

The special features of this model are as follows:

i. This is most sensitive to the variations in the degree of slope steepness, whereas USLE is most sensitive to change in the value of factor ‘C’.

ii. Change in slope steepness and slope length affects the prediction of soil loss by both the models, equally.

iii. Particularly, at low sensitivity level the use of Stehlik model has more importance to change in the rainfall factor, and less importance to change in the soil erodibility. This should always be kept in view for calculation of soil loss.

Various factors involved in this model are defined as under:

i. Climatic Factor (D):

It is expressed in terms of precipitation falling at the intensity equal to or greater than √5t, in which t is the rainfall duration (minute). The values of climatic factor (D) for Czechoslovakia varies between 0.26 and 0.82, in which the lower value is used for low lands and higher one is for mountainous regions. The value of ‘D’ can also be computed based on the mean precipitation (R), using following equation –

D = 0.0014 R – 0.38 … (21.50)

In which, R is the mean annual precipitation. However, Zachar (1982) has reported the values of climatic factor (D) for precipitations ranging from 10 to 60 minutes duration at different frequencies, as cited in Table 21.23.

ii. Petrological Factor (G):

It is also nomenclatured as the geological factor, used to assess the distribution of various rocks according to the resistance and permeability of their weathered debris, which ultimately make effect on runoff and soil loss. The values of G as per permeability of rock and granulation of weathered debris are given in Table 21.24.

iii. Erodibility of Soil (P):

Sometimes, it is also referred as soil factor, which expresses the erodibility of the soil. The value of P is determined based on the percentage soil particles of diameter less than 0.1 mm and organic content present in the soil. The P values for different soil characteristics are shown in Table 21.25.

iv. Slope Steepness (S):

The slope steepness or slope factor is expressed in terms of soil surface gradient according to the following relationship –

S = 0.24 + 0.106 s + 0.0028 s² … (21.51)

In which, S is the slope factor and s is the slope gradient (%). If slope gradient of the field is known, then value of S can be computed. However, the values of S for different slope gradients (s) are also cited in Table 21.26.

The table value indicates that the outcome of calculation of soil loss using Stehlik model varies significantly with the slope gradient. As per table 21.26 if slope gradient increases from 5 to 50% then value of slope factor gets increase about 34.3 times greater to that of 5% gradient.

v. Slope Length Factor (L):

It is determined on the basis of slope length. For different slope lengths the values of L are given in table 21.27.

From the table value, it is evident that the value of L increases about 1.5 times as the slope length becomes double, (i.e. from 20 m to 40 m)

vi. Vegetation Factor (O):

The vegetation factor is dependent on the percentage soil cover due to particular vegetation. Its values are given in Table 21.28.

Soil Loss Prediction:

The estimation procedure of soil loss, using Stehlik model is similar to the Universal Soil Loss Equation.

5. Slemsa Model:

The Soil Loss Estimation Model for South Africa (SLEMSA) developed by Elwell (1978) is a new approach to soil loss estimation, found suitable particularly for those countries which are unable to support expensive research programes on soil loss, but urgently require a decision-making aid to combat soil loss. This model has been designed to predict mean annual soil loss, caused by sheet erosion in the arable land between two adjacent contour ridges.

It overcomes various disadvantages encountered in the use of Universal Soil Loss Equation (i.e. USLE), such as:

i. The evaluation of involved factors from soil loss measurements under field test.

ii. The construction of plots for experiment is expensive; and the experiments must be for about 30-years, to obtain a reasonable estimate of the mean soil loss.

iii. A lot of plots are required to measure the soil loss for every possible combinations of climate, soil, crop, slope and tillage and management practices.

iv. There is large variations in agro-climatic characteristics between America and Africa, hence there will be unsuitable to transfer the hydrologic data. Due to this reason, it is always advised to test the validity of USLE before going to make its use.

v. Furthermore, the farming methods and practices followed are also quite different in both the countries, therefore, it is inappropriate to use the values of USLE factors to the other conditions.

vi. To estimate satisfactory value of soil loss using USLE, a long-term data collection programme is essentially required.

The above points make unsuitable to use the USLE for Africa or other countries. The SLEMSA model offers advantages for use of scarce data.

6. Erosion Deposition Process Model:

Full description of this model is given by Rose et. al. (1983 b, c, d). The model is developed by relating the sediment flux at any position on a plane and at any lime by runoff event to the factor on which this sediment flux depends. The sediment flux (q_s) is defined as the mass of sediment flowing per unit time across a unit stream width, perpendicular to the direction of flux.

Erosion and Deposition Process:

This model considers following three processes, which affect the sediment concentration, excluding the situation of land slides or gullies formation –

(a) Rainfall Detachment:

It is the process in which raindrop splashes the sediment from the soil surface into the overland flow. The rate of rainfall detachment is given by the following equation –

e = a . C_e .P² … (21.59)

Where,

e = rate of particles detachment (kg/m²/s)

a = measure of soil detachability by rainfall

C_e = fraction of soil surface exposed to the raindrop

P = rainfall intensity

(b) Sediment Deposition:

It is the process in which sediments are settle down under the action of gravity force. The rate of sediment deposition depends on the particle size distribution. In case of sands it takes place at rapid speed, whereas for clay-size aggregates or particles, it is being very slow. It is –

(c) Rate of Sediment Entrainment:

It is the process by which the overland flow picks up the sediments from the soil surface, whether it is rill, between the rills or the sheet flow without rills. The onset of entrainment can correspond the transition from dominantly laminar to turbulent flow as described by Turner et. al (1985). The rate of sediment entrainment (r) in overland flow has similarity to the bed load in streams.

It has been shown that, the rate of bed load transport can be related to the excess of ‘stream power’ (Ω) above threshold value (Ω_o) required to entrain the sediment. The ‘stream power’ is defined as the rate of working of shear stress between the sediment and the stream bed, which is expressed as Ω = ρ . g . S . R₁ . x.

The sediment entrainment rate (r) can be determined by developing an analogue approach, using mass conservation of sediment in the elementary section of the overland flow, shown in Fig. 21.11. The developed equation can be used for computing the sediment entrainment (r). However, a fraction of unprotected soil surface ‘C_r‘ from entrainment by the overland flow is there, which plays similar role as C_e in equation 21.59.

As for as calculation of ‘stream power’ is concerned, it can be done based on the bed slope and water flux (q) which is the rate of volume of water flow per unit strip width (m²/s). The stream power is the maximum rate at which the energy available per unit area, is not totally used for entraining and transporting the sediment.

Model of Erosion/Deposition on a Plane:

The model is developed on the basis of mass conservation of sediment in the elementary section of overland flow, as shown in Fig. 21.11 along with the relationship of sediment concentration theories and hydrology. The mass conservation of sediment class i and C_i can be presented by the following equation –

In which, D is the depth of overland flow at any time and position of the plane. In this equation, the right hand side part represents the net erosion rate with equation 21.59 suitably modified for e_i; equation 21.60 for d_i and a more complex expression for r_i. For a good approximation the partial differential equation 21.61 can be reduced to an ordinary (1st order) differential equation, which can be easily solved. The solution of equation 21.61 is given as –

Where,

C (L, t) = sediment concentration at the bottom of the plane of length L as the function of time t, expressed as kg/m³.

I = number of sediment class.

r_i = 1 + V_i/Q

ρ = density of water, taken as 1000 kg/m³

g = acceleration due to gravity, 9.81 m/s²

S = land slope, counted as sin of indication angle.

K = 0.276 ƞ

ƞ = efficiency of net sediment entrainment and transport

C_r = fraction of soil surface, unprotected from entrainment by the overland flow

x* = the distance at downslope from the top of the plane beyond which entrainment of sediment commences. It is related to Ω_o by the following equation, given by Rose et al (1983 c).

7. Simplified Erosion Process Model:

The model (i.e. equation 21.62) can be simplified as under –

The equation 21.62 is –

In which,

A = net contribution of sediment load from rainfall detachment over deposition, and

B = net contribution of entrainment over deposition.

In equation 21.64 the term A is very small as compared to the term B, because greater the value of ‘Q’ the larger will be sedimentary unit and so larger r_i. Therefore, neglecting the term A from equation

a = 2.884 – 8.1209 F

b = 0.4681 + 0.7663 F

In equation 21.65 the entrainment efficiency ƞ is only time variable. Assuming this term as the average value of soil erosion, the sediment concentration can be taken as constant for a particular erosion event, and can be written as –

This simplified model is suitable for interpreting the experimentation data on soil erosion and for the design and assessment of soil-conserving management practices for agriculture at any location and for any scale and type of cultivation.

8. Water Erosion Prediction Project (WEPP) Model:

WEPP is widely used physically process-based erosion model. It was developed on the basis of system modeling approach for predicting the soil loss and selecting catchment management practices for soil conservation. The basic erosion and deposition equations in WEPP are based on the mass balance that uses rill and inter-rill concept of soil erosion, which is a steady-state sediment continuity equation. The WEPP model computes erosion by rill and inter-rill processes. The sediment delivery to rill from inter-rill area is computed by using the following equation –

Di = Di .Ie² .Ge .Ce .Sf … (21.71)

Where,

Di = sediment delivery from the inter-rill area to the rill (kg/m²/s)

Ki = inter-rill erodibility (kg/m⁴/s)

Ie = effective rainfall intensity (m/s)

Ge = ground cover adjustment factor and

Sf = slope adjustment factor, is calculated using the following formula –

Sf = 1.05 – 0.85 exp (–4 sin α)

In which, α is the slope of soil surface towards rill. The rill erosion is estimated by using the following formula –

Dc = Kr. (T – Tc) … (21.72)

in which, Kr is the rill erodibility (sec/m); T is the hydraulic shear of flowing water (Pa) and Tc is the critical hydraulic shear that must be sufficient before occurring the rill detachment (Pa).

9. REGEM Model for Prediction of Gully Erosion:

The REGEM model predicts the soil erosion from an ephemeral gully. The involved computations under this model follow the following formulae –

Time Base of a Triangular Hydrograph:

It is computed based on the peak discharge (Q_p) and runoff volume (V_b) at the mouth of ephemeral gully. The following formula is used –

Prediction of Raindrop Induced Soil Erosion:

It can be suitably computed by using USLE, WEPP model and EUROSUM. In WEPP model the erosion component is based on the rills and inter-rill areas. The sediment yield is from the inter-rill areas. The erosion equation of WEPP model represents steady state sediment continuity equation. The equation is given as below –

10. Eurosum Model:

The European Soil Erosion Model (EUROSUM) is developed to estimate the sediment transport, erosion and deposition over the land surface by rill and inter-rill possesses in the fields and small catchments. This is single event based model. It can be used to simulate dynamic behavior of raindrop even within the storm. EROSUM involves the water and sediment routing structure.

In EUROSUM the traditional concepts of rills and inter-rill processes are not followed. On the other hand, the raindrop and flow processes are considered for all the areas with the distinction between rills and inter-rill areas being one of the geometry. The rills are considered as trapezoidal channel; and inter-rill areas as the surface without roughness. The equation for determining the detachment of soil particles by rainfall is given as below –

in which, k is the index of soil particle’s detachability, is determined by conducting experiment; ρ_s is the particles density; KE is the kinetic energy of raindrop impacting the soil surface; z is an exponent depends on the soil texture; and h is the mean depth of water flow over soil surface. The particle detachment by flow is modeled as under – 

In which, β is the flow detachment efficiency factor; w is the width of flow; v_s is the settling velocity of soil particle; T_c is the transport capacity of flow. The value of T_c for rill flows can be determined by using the relationship developed by Govers (1990); and inter-rill flow can be predicted by the relationship developed by Everaert (1991).

11. Kinros Model:

Woolhisar et. al. (1990) developed the KINROS model for predicting the soil erosion, based on the assumption that the land surface in watershed is as the series of inter-linked plane with uniform slope. It computes the soil loss by determining the rate of sediment flow from a given point in a given time. Model is based on the mass balance equation, given as under –

in which, C is the sediment concentration; A is the cross sectional area of the flow; Q is the rate of flow discharge; DR is the rate of particle detachment by raindrop; DF is the net rate of particle detachment by flow (its value is taken +ve for detachment and -ve for deposition); q_s is the external input or extraction of sediment per unit length of flow; x is the horizontal distance and t is the time.